Trigonometric Ratios of $30^{\circ}, 45^{\circ}$ and $60^{\circ}$
| $\begin{array}{|c|c|c|c|c|c|c|} \hline \theta & \sin \theta & \cos \theta & \tan \theta & \cot \theta & \sec \theta & \csc \theta \\ \hline 30^{\circ}\left(\displaystyle\frac{\pi}{6}\right) & \displaystyle\frac{1}{2} & \displaystyle\frac{\sqrt{3}}{2} & \displaystyle\frac{\sqrt{3}}{3} & \sqrt{3} & \displaystyle\frac{2 \sqrt{3}}{3} & 2 \\ \hline 45^{\circ}\left(\displaystyle\frac{\pi}{4}\right) & \displaystyle\frac{\sqrt{2}}{2} & \displaystyle\frac{\sqrt{2}}{2} & 1 & 1 & \sqrt{2} & \sqrt{2} \\ \hline 60^{\circ}\left(\displaystyle\frac{\pi}{3}\right) & \displaystyle\frac{\sqrt{3}}{2} & \displaystyle\frac{1}{2} & \sqrt{3} & \displaystyle\frac{\sqrt{3}}{3} & 2 & \displaystyle\frac{2 \sqrt{3}}{3} \\ \hline \end{array}$ |
|---|
Exercise (10.4)
- Draw a right triangle and find $\angle A$.
(a) $\sin A=\displaystyle\frac{1}{2}$
(b) $\cos A=\displaystyle\frac{\sqrt{3}}{2}$
(c) $\tan A=\sqrt{3}$
(d) $\cot A=1$
(e) $\sec A=\sqrt{2}$
(f) $\csc A=2$ - $\angle A=30^{\circ}, \quad c=30$, find $a$.
- $\angle A=60^{\circ}, \quad a=15$, find $b$.
- $\angle B=45^{\circ}, \quad a=16$, find $c$.
- $\angle B=30^{\circ}, \quad b=8$, find $c$.
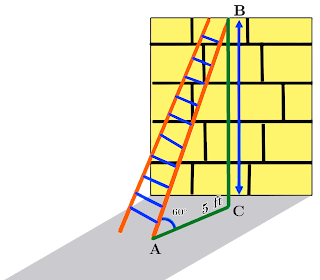
- A ladder is placed along a wall such that it upper end is touching the top of the wall. The foot of the ladder is $5\ \text{ft}$ away from the wall and the ladder is making an angle of $60^{\circ}$ with the level of the ground. Find the height of the wall.
- $\cot ^{3} 45^{\circ}+4 \sin ^{3} 30^{\circ}$.
- $\tan 60^{\circ} \cot 30^{\circ}+4 \sec ^{2} 30^{\circ}$
- $\tan ^{2} 45^{\circ}+\sin 30^{\circ}-\cos ^{2} 30^{\circ}+2 \tan ^{2} 60^{\circ}$.
- $\displaystyle\frac{1}{2} \sec ^{2} 30^{\circ}+\csc ^{2} 45^{\circ}-2 \tan ^{2} 30^{\circ}$.
For each of the right triangles $A B C$, find the indicated sides.
Find the numerical value of:
စာဖတ်သူ၏ အမြင်ကို လေးစားစွာစောင့်မျှော်လျက်!